Nonlinear inequalities may seem more complicated and difficult to solve than linear inequalities. However it is not really the case. There is one simple way to solve a nonlinear inequality. It’s called the test point method. I will explain this with an example.
Example. Solve the inequality $x^2\leq 5x-6$.
Solution. The inequality can be rewritten $x^2-5x+6\leq 0$. First we find points at which $x^2-5x+6=0$. Since $x^2-5x+6=(x-2)(x-3)$, $x=2,3$. These two points divide the real line into 3 regions, where $x<2$, where $2<x<3$, and where $x>3$ as shown in Figure 1.

Figure 1. Quadratic Inequality
In each region we pick a test point to see if that test number satisfies the given inequality. If it does, any other number in the same region would satisfy the inequality. If not, any other number in the same region wouldn’t either. While this is pretty cool, you may wonder why this works. One number speaks for the entire numbers in the same region. It’s hard to explain here though but it is due to the continuity of the function $f(x)=x^2-5x+6$. I will leave it at that and will not delve into that any further. You will understand what I said when you learn calculus. In the region $x<2$, I would pick $x=0$ for a test point. But $x=0$ won’t satisfy the inequality as the LHS is $6>0$. Move onto the next region $2<x<3$. I pick $x=2.5=\frac{5}{2}$. Since $\left(\frac{5}{2}\right)^2-5\frac{5}{2}+6=\frac{25-50+24}{4}=-\frac{1}{4}<0$. So this means that $2<x<3$ is a solution of the inequality. In the final region $x>3$ I pick $x=4$. $(4)^2-5(4)+6=2>0$ so no number in this region would satisfy the inequality. Since $x=2$ and $x=3$ also satisfy the inequality, the overall solution is $2\leq x\leq 3$ or $[2,3]$ in interval notation.
The inequalities like one we just did is called quadratic inequalities. For quadratic inequalities we can actually classify solutions depending on inequalities without going through the test point method every time. Let us assume that the quadratic function $f(x)=ax^2+bx+c$ with $a>0$ has two real solutions $\alpha$ and $\beta$ ($\alpha<\beta$). Then the graph of $f(x)$ would look like one in Figure 2. You can find the solution of each of the following quadratic inequalities easily from the graph in Figure 2.
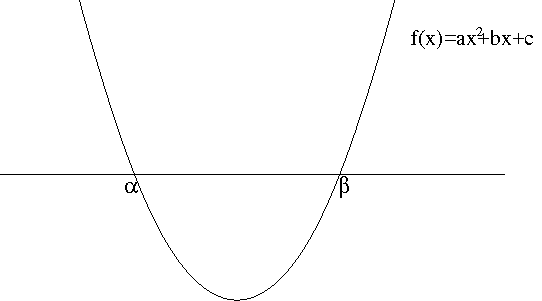
Figure 2. Quadratic Inequality
- $ax^2+bx+c>0$: $x<\alpha$ or $x>\beta$. In interval notation, $(-\infty,\alpha)\cup(\beta,\infty)$.
- $ax^2+bx+c\geq 0$: $x\leq\alpha$ or $x\geq\beta$. In interval notation, $(-\infty,\alpha]\cup[\beta,\infty)$.
- $ax^2+bx+c<0$: $\alpha<x<\beta$. In interval notation, $(\alpha,\beta)$.
- $ax^2+bx+c\leq 0$: $\alpha\leq x\leq\beta$. In interval notation, $[\alpha,\beta]$.
Let us go over a couple more examples of nonlinear inequalities that are not quadratic inequalities.
Example. Solve $x(x-1)^2(x-3)<0$.
Solution. The method is the same as the first example. We use the test point method. First find $x$ at which $x(x-1)^2(x-3)=0$. They are $x=0, 1, 3$. So there are 4 regions under consideration. $x<0$, $0<x<1$, $1<x<3$, and $x>3$. In the region where $x<0$, the test point $x=-1$ results the sign of the LHS is $+$. So $x<0$ is not a solution. In the region $0<x<1$, the test point $x=\frac{1}{2}$ results the sign of the LHS $-$, so $0<x<1$ is a solution. In the region $1<x<3$, the test point $x=2$ results the sign of the LHS still $-$. This is actually due to $(x-1)^2$. In general if you see an even number of repeated term $x-a$ in your polynomial inequality like the one we have the sign of the polynomial does not change at $x=a$. A little goody to know so you can save time. Let us move onto next and last one. For $x>3$ the test point $x=4$ results the sign of the LHS $+$, so $x>3$ is not a solution. Therefore, the overall solution is $0<x<1$ or $1<x<3$. In interval notation, it is $(0,1)\cup(1,3)$.
Example. Solve $\frac{1+x}{1-x}\geq 1$.
Solution. First rewrite the inequality as $\frac{1+x}{1-x}-1\geq 0$ which simplifies to $\frac{2x}{1-x}\geq 0$. Inequality like this we consider points at which the numerator is 0 and also points at which the denominator is 0. In our case they are $x=0, 1$ and these two points divide the real line into three regions: $x<0$, $0<x<1$, $x>1$. In the region $x<0$, the test point $x=-1$ results the sign of the LHS $-$, so $x<0$ is not a solution. In the region $0<x<1$, the test point $x=\frac{1}{2}$ results the sign of the LHS $+$, so $0<x<1$ is a solution. Finally in the region $x>1$ the test point $x=2$ results the sign of the LHS $-$, so $x>1$ is not a solution. Since $x=0$ also satisfies the inequality, the overall solution is $0\leq x<1$ or $[0,1)$ in interval notation.