This is a guest post by Dr. Lawrence R. Mead. Dr. Mead is a Professor Emiritus of Physics at the University of Southern Mississippi.

It is often stated in elementary books and repeated by many that the reason that an object with mass cannot achieve or exceed the speed of light in vacuum is the “mass becomes infinite”, or “time stops” or even “the object has zero size”. There are correct viewpoints for why matter or energy or any signal of any kind cannot exceed light speed and these reasons have little to do directly with mass changes or time dilations or Lorentz contractions.

Reason Number One

Consider a signal of any kind (mass, energy or just information) which travels at speed $u=\alpha c$ beginning at point $x_A$ at time $t=0$ and arriving at position $x_B$ at later time $t>0$. From elementary kinematics,
$$t=(x_B -x_A)/u = {\Delta x \over \alpha c}.$$
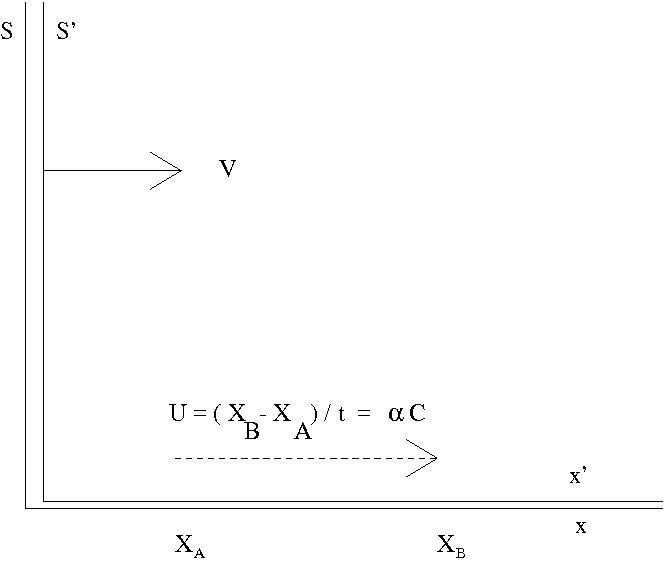Now suppose the signal travels at a speed exceeding $c$, that is $\alpha > 1$. Let us calculate the elapsed time as measured by a frame going by at speed $V<c$. According to the Lorentz transformation,
\begin{equation}\label{eqno1}t’ = \gamma (t-{Vx \over c^2}),\end{equation} where $\gamma=\frac{1}{\sqrt{1-\frac{V^2}{c^2}}}$.
There are two events: the signal leaves $x=x_A$ at $t=0$, and the signal arrives at $x=x_B$ at time $t=\Delta t$. According to \eqref{eqno1}, these events in the moving frame happen at times,
$$t’_A=\gamma ( 0 -Vx_A/c^2)$$ and
$$t’_B=\gamma (\Delta t – Vx_B/c^2).$$
Thus, the interval between events in the moving frame is, \begin{equation}\begin{aligned}\Delta t’ &= t’_B-t’_A\\
&=\gamma \Delta t -\gamma \frac{V}{c^2}(x_B-x_A)\\
&=\gamma \Delta t ( 1-\alpha V/ c).\end{aligned}\label{eqno2}\end{equation}
Now suppose that $\alpha V/c > 1$, which implies that,
$$ c > V > c/\alpha .$$ Then for moving frames within that range of speeds it follows from \eqref{eqno2} that,
$$\Delta t’= t’_B-t’_A <0,$$ meaning physically that the signal arrived before it was sent! This is a logical paradox which is impossible on physical grounds; no one will argue that in any frame a person can be shot before the gun is fired, or you obtain the knowledge of the outcome of a horse race before the race has begun.

Well now what if the two events at $x_A$ and $x_B$ are not causally connected but one (say at $x_B$ for definiteness) simply happened after the other? How does the above argument change? How does the above math “know” that there is or is not a causal connection between the events? Everything goes the same up to the second line of equation \eqref{eqno2}:
\begin{equation}\label{eqno3} \Delta t’ = \gamma \{ \Delta t – V(x_B-x_A)/c^2 \}. \end{equation}
Can there be a moving frame of speed $V<c$ for which the event at $x_B$ (the later one in S) happens before the event at $x_A$ (the earlier one in S)? If so, $\Delta t’ < 0$; from \eqref{eqno3} then we find,
$$\Delta t – V(x_B-x_A)/c^2 < 0, $$ or solving for $V$,
$$ c > V > c{c\over \Delta x/ \Delta t}.$$ In order for $V$ to be less
than $c$, it must therefore be that, ${c\over \Delta x /\Delta t} < 1$, or
$${\Delta x \over \Delta t} > c.$$ This is possible for sufficiently large $\Delta x$ and/or sufficiently small $\Delta t$ because the ratio ${\Delta x \over \Delta t}$ is not the velocity of any signal, though it has the units of speed.

What is the Speed of “Light” Anyway?

Note that the Lorentz transformation contains the speed $c$ in it. What is this speed? Without referencing Maxwell’s equations of Electromagnetism, one does not know that $c$ is in fact the speed of light itself. But the above analysis shows – without reference to Maxwell – that the speed $c$ cannot be exceeded. And what is the speed talked about in the previous discussion? Well, it is the maximum speed at which one event can influence another with given (fixed) separation – thus, $c$ above isn’t really the speed of light at all; rather it is the speed of causality!

Reason Number Two

Imagine, for example, a constant force $F$ acting on a particle of (rest) mass $m$. Newton’s second Law in its relativistic form gives,
\begin{equation}\begin{aligned} F &= {dp \over dt} \\
&= {d \over dt} \, mv\gamma \\
&= m \gamma^3 \, \dot v, \end{aligned}\label{eqno4}\end{equation}
where we have assumed straight line motion. This is an autonomous differential equation whose solution, assuming the object is initially at rest, is,
$$ v(t)=at/(1+a^2t^2/c^2)^{1/2}, $$
where $a=F/m$. It is clear that as $t \to \infty$, $v(t)$
approaches $c$ and not infinity. Moreover, the differential impulse at arbitrary time $t$ on the particle can be found from taking the derivative of $v(t)$ given in the last equation,
\begin{equation}\label{eqno5}m\, dv = { F \, dt \over (1+a^2t^2/c^2)^{3/2}}. \end{equation}
From equation \eqref{eqno5}, it is clear that the incremental speed increase $dv$ over time $dt$ approaches zero as $t \to \infty$. Thus, from this point of view we see that while the force still does work, the increase in speed for a given interval of time and incremental amount of work, is less and less as time goes on which is why the speed never reaches $c$ over any finite time interval.

Reason Number Three

In the interval of time $dt$ as measured in some inertial frame observing a moving body, the clock attached to the body ticks off proper time
\begin{equation}\label{eqno6}d\tau = \sqrt{1-v^2/c^2}\, dt. \end{equation}
However, for light $v\equiv c$, and therefore $d\tau\equiv 0$. Light takes no proper time to go between two points however distantly separated in space. Thus, no object could travel faster than taking no time. This is the oft-repeated mantra of textbooks, and, while the mathematics verifies it, there are far more fundamental reasons, the best being causality as outlined above.