I will be more than happy to be corrected if I am wrong, but, as far as I am aware, there is no confirmed FTL (Faster-Than-Light) phenomenon. In the past, there were some reported FTL phenomena with the speed of quasars and neutrinos. It turns out the alleged FTL speed of quasars was due to an optical illusion and FTL neutrino anomaly was caused by a faulty equipment set-up. If an FTL phenomenon exists, it would violate special relativity unless it is a detection of tachyons whose initial speed already exceeds the speed of light. This may sound strange and self-contradictory but even if we detect tachyons, they may actually not FTL particles contrary to our observations. Here, I would like to offer how such a possibility may occur.
Let us start with our universe with the Minkowski metric $ds^2=-dt^2+dx^2+dy^2+dz^2$. For the sake of simplicity, we assume here that $c=1$. The Minkowski plane can be identified with split-complex plane via the map: $(t,x)\mapsto t+xj$, where $j^2=1$. In fact, the identification is an isometry: If $\zeta=t+xj$, then $-t^2+x^2=-\zeta\bar\zeta$. The map $\zeta=t+xj\mapsto j\zeta=x+tj$ gives rise to the reflection of $\zeta$ about the light ray $t=x$.
Applying this reflection on the Minkowski metric results in a different universe with new metric $ds^2=dt^2-dx^2+dy^2+dz^2$. Figure 1 shows the light cone of our universe (in blue) and that of its counterpart (in red).
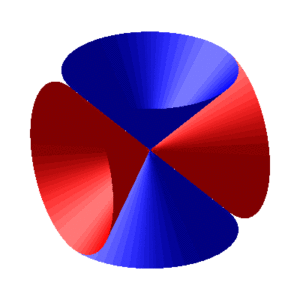
Figure 1: The Light Cone of Our Universe (in Blue) and That of Its Counterpart Universe (in Red) with z-Coordinate Suppressed
As you can see in Figure 1, spacelike vectors in our universe fall into the timelike region of the other universe. This means that what we observe as a superluminal motion in our universe could be a subluminal motion in the other universe. To see that more clearly, let us consider a rotation (Lorentz boost) in the Minkowski plane of the other universe. The new rotated coordinates $(x’,t’)$ are given in terms of the original coordinates $(x,t)$ by
\begin{align*}
x’&=\cosh\phi x-\sinh\phi t\\
t’&=-\sinh\phi x+\cosh\phi t
\end{align*}
From the second equation, we see that $x’$-axis ($t’=0$) is moving at a constant speed $$v=\frac{t}{x}=\frac{\sinh\phi}{\cosh\phi}=\tanh\phi$$ In their universe, the theory of special relativity must hold as well. This means that $v=\tanh\phi<1$. This motion in the other universe would, however, be observed as a superluminal motion in our universe because in our universe the speed of $x’$-axis is $$v=\frac{x}{t}=\frac{\cosh\phi}{\sinh\phi}=\coth\phi>1$$
There is more to this other universe. I have been considering quantum mechanics based on split-complex numbers instead of complex numbers. In this model of quantum mechanics, antiparticles are described by the reflection of wave functions that describe particles about the light ray $t=x$ in the Minkowski plane. (In conventional quantum mechanics, antiparticles do not show up at non-relativistic level.) These reflected wave functions have negative probabilities which deem unphysical. However, if one assumes the existence of the other universe, their probabilities turn out to be positive in that universe. It would be logical to assume that in the beginning, the same amount of particles and antiparticles were created. But there aren’t as many antiparticles as particles in the universe. This is called Baryon Asymmetry. In light of the quantum mechanics model based on split-complex numbers, here is a possible scenario. The Big Bang created not just one, but two twin universes, one with signature $(-+++)$ which is made mostly of matter and the other with signature $(+-++)$ which is mostly made of antimatter. These two universes are separated by their respective light cones. In other words, the light cone of each universe is its boundary. Unless one travels faster than the speed of light, he cannot cross over to the other universe. Of course, we know that the theory of special relativity prohibits this. And even if he somehow succeeds crossing over to the other side, he is doomed to perish to energy as soon as he crosses over.
